- #FINDING SOLUTIONS FOR 3 EQUATION SYSTEMS WITH 2 VARIABLES HOW TO#
- #FINDING SOLUTIONS FOR 3 EQUATION SYSTEMS WITH 2 VARIABLES FREE#
For each system, choose the best description of its solution. Two systems of equations are given below. Solving a 2x2 system of linear equations that is inconsistent or.
Solving a 2x2 system of linear equations that is inconsistent or. Infinitely Many Solutions (c) Is A invertible? (b) What can you say about the solution(s) to the linear system Az = ? A. Let A be the 3 x 3 matrix: A= 3 3 0 -4 1-3 5 1 (a) Find det(A) by hand. (2 points) Suppose the linear system Ac = b has a partitioned matrix that has been reduced 1 1 1 1 0 0 1 1 to 0 0 0 1 Which of the following describes the nature of the solution set of the 0 0 0 0 0 0 0 0 0 0 system? a) no solution b).ġ 2. 
Please explain why the answer is the answerĢ. Help! I need help with this linear algebra question I had got
help! I need help with this linear algebra question I had got. (1) Consider a CONSISTENT system(defined over R) of. either no solution or infinitely solutions #FINDING SOLUTIONS FOR 3 EQUATION SYSTEMS WITH 2 VARIABLES FREE#
infinitely many solutions with one free variable E. infinitely many solutions with three free variables D. If the definitely true? rank of the coefficient matrix is 4, which of the following statements is A. (1) Consider a CONSISTENT system(defined over R) of 7 linear equations in 5 variables.
#FINDING SOLUTIONS FOR 3 EQUATION SYSTEMS WITH 2 VARIABLES HOW TO#
I don't understand how to get the answer for this question. (1) Consider a CONSISTENT system(defined.
I don't understand how to get the answer for this question. System were nonhomogeneous, how many solutions might there be?ĭetermine the value of k such that the system of linear equations has infinitely.ĭetermine the value of k such that the system of linear equations has infinitely many solutions.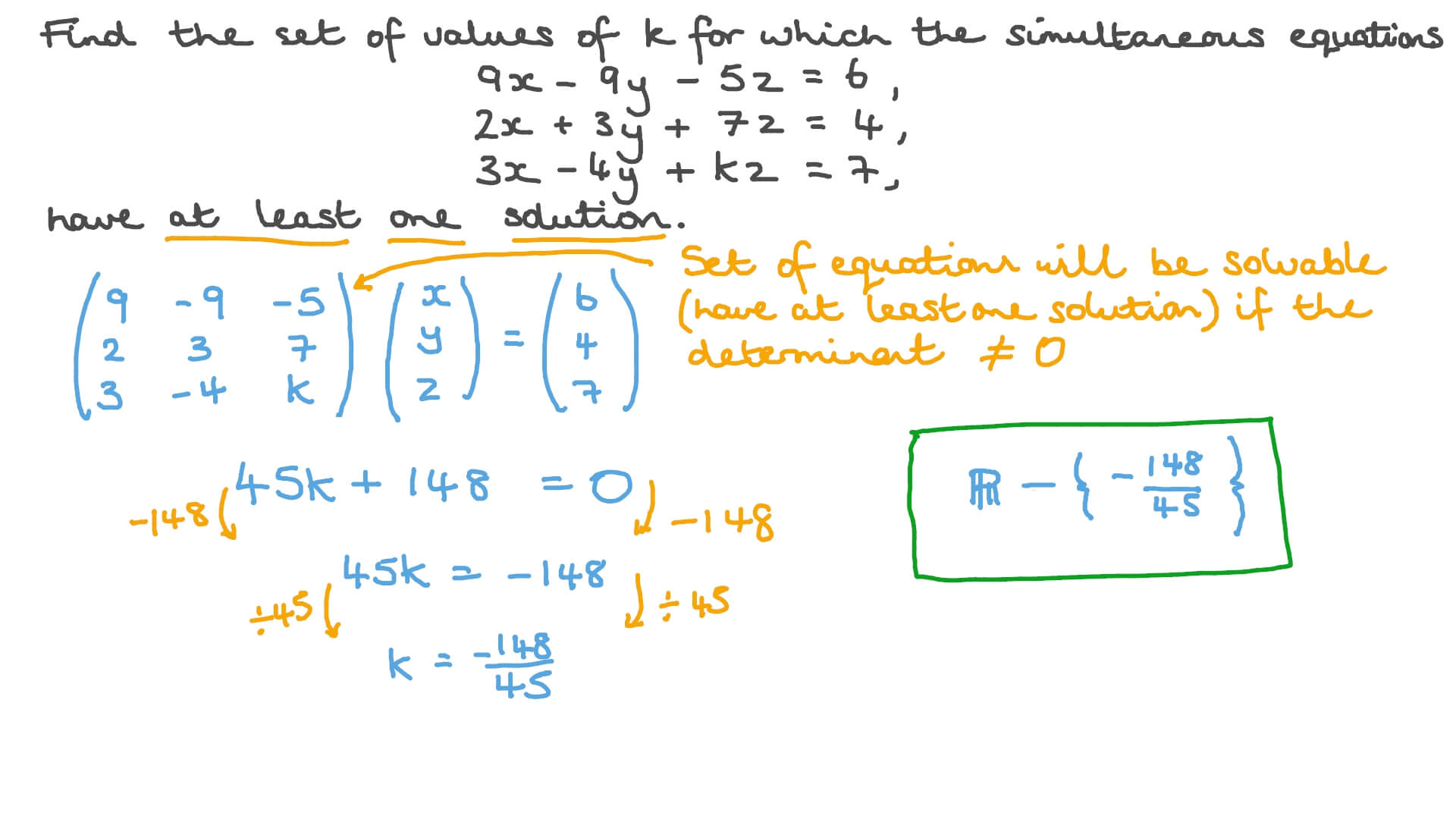 
Geometrically, why does a homogenous system of two linearĮquations in three variables have infinitely many solutions? If the
Geometrically, why does a homogenous system of two linearĮquations in three variables have infinitely many. The system has 0 0 -1 2 -3 a) a unique solution b) no solutions c) infinitely many solutions with one free variable d) infinitely many solutions with two variables e) infinitely many solutions with three variables Consider the following augmented matrix of a system of linear equations: [1 1 -2 2 3 1 2 -2 2 3 0 0 1 -1 3. Consider the following augmented matrix of a system of linear equations: [1 1 -2 2.ġ. Classify the equations as a conditional equation, an identity, or a contradiction and then state the solution.40013 1 2 3 14 Example of linear equations with unique Solution Suppose there is an Augmented Matrix with a 3x3 Identity Matrix on the right A=lioli Now performing any number of Elementary now operations on this kind of Matrix gives a system af linear equations with unique solution x=1, y = 2 z=3 one of them for example is T i l the equation system is x + y + z = 6 x+2y +32=14 hus unique solution x+y -2= Example of a system of equations with Infinitely many Solutions 113 114 1 is an augmented Matrix with Last To -1361-8 To oolol row completely zero If we perform any number of elementary row operations on this kind of a Matrix we get Systems with Infinite Solutions z=t, free variable x and y can be expressed in term of t one of the examples is 113-14 x+3y-2=4 14 -1 28 22 -7 ulo 2x - 2y +48=0 has infinite Solutions Example of Systems with no Solutions The Augmented Matrix of the following form Containing Zeroes all to the right and Non zero at the right 113-1141 After any finite row operations gives a System ooolaz) with no Solutions one of the example is (13-1 la 4-1 28 x+3y-2=4 4x - y + 2y = 8 O -13 6 -8 12-7 u 1-3 uz-y +23=8 has no solutions 24-7y +43 = -3
0 kommentar(er)
